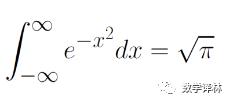Author | Xi Nanhua
Source | Mathematical Translation Forest
Quantity and shape are the basic attributes of matter and things. They are the objects of mathematical research, which determines the value and significance of mathematics.
Mathematics is actually concerned with the mathematical laws of quantity and shape, which is a reflection of the real world. The law of mathematics is the law of the basic attributes of matter and things, and it is the most essential part of the laws of nature and society.
The meaning and value of mathematics seems needless to say, but the language of mathematics is abstract, and the abstract face is basically that people don’t like it, and it is often mistaken for being far away from the real world and human fireworks, which is quite unjust. The abstract value will be mentioned later.
1. What was mathematics like in the distant past?
Mathematics has a long history. It is generally believed that mathematics, as an independent and theoretical discipline, appeared between 600 BC and 300 BC, and Euclid’s Elements (about 300 BC) is a brilliant model.
It systematically sorts out the mathematical achievements of ancient Greeks by using axiomatic system, and its system, the expression of mathematical theory and the way of thinking embodied in the book have far-reaching influence on the development of mathematics and even science. Throughout the history of mathematics development, The Original is the most influential mathematics book.
Another great mathematical work in ancient Greece was apollonius’s "Conic Curve", which was later than "The Original" in time. In addition to synthesizing the achievements of predecessors, this book has unique innovation, excellent material organization and flexible writing. This book can be called the pinnacle of conic curve, and later generations can hardly say anything new on this subject, at least geometrically.
Almost at the same time, there was a study of the history of mathematics. Eudemus (about 370-300 BC), a student of Aristotle (384-322 BC), wrote books on the history of mathematics.
The history of human civilization is much longer. About 10 thousand years ago, human beings began to settle in a region and live by agriculture and animal husbandry. Writing appeared much later, around 3200 BC. Before that, the progress of human beings in mathematics was extremely slow, because the level of development was low, the demand for mathematics was extremely low, and it was very difficult to form abstract mathematical concepts from scratch.
The most basic concepts of mathematics, numbers and straight lines, took a long time to form.
At first, people’s concept of logarithm was associated with specific objects, such as a tree, a stone, two people, two fish, and so on. Time, constantly passing, … Gradually, people realized the common numerical attribute of a tree, a stone and other concrete objects, and the abstract concept of number was formed.
Similarly, at first, people’s concept of line was associated with specific line shapes such as trees, branches, ropes and edges of objects. Time goes by … gradually, people realize the common shape attributes of concrete objects such as straight trees, taut ropes and straight edges of some objects, and the abstract concept of straight lines is formed.
The formation of the concepts of number and straight line is a leap for human beings to understand nature.
The emergence and development of mathematics is driven by real life. Arithmetic and geometry were first produced.
Realistic needs have led to the calculation between numbers (such as distributing food, exchanging goods, the number of days before the specified date, etc.). So you need to give the number a name and write it down and tell others.
Digital symbols introduced from the beginning of writing have played a great role in the development of arithmetic.
This is the first step to introduce general mathematical symbols and formulas. In the next step, the introduction of arithmetic operation symbols and unknown symbols was completed very late, and it was constantly improved. For example, the familiar symbols of addition, subtraction, multiplication and division were not used until the 15th and 18th centuries.
Arithmetic was first developed in Babylon and Egypt, due to the practical needs of taxation, land measurement, trade, architecture, astronomy and so on. But here is mainly for the calculation and answer of specific problems. This form of arithmetic is not a mathematical theory, because there is no general property (or law) about numbers.
The transition to theoretical arithmetic is gradual. Ancient China, Babylonia and Egypt already knew the possibility of millions. Here has shown the possibility of infinite continuation of the series. But people didn’t realize this clearly soon.
Archimedes (287-212 BC) pointed out the method of naming the number of a large number of sand grains in the Sand Counting Law. This is a matter that needs to be explained in detail at that time. It’s not an easy thing today.
In the third century BC, the Greeks clearly realized two important ideas: the sequence of numbers can continue indefinitely; We can not only use specific numbers, but also discuss general numbers, and establish and prove the general properties of numbers.
For example, there are infinitely many proofs of prime numbers in the elements, and this conclusion and proof will be mentioned later. Arithmetic thus developed into theoretical arithmetic.
Theoretical arithmetic is actually the theory of numbers, and the calculation of specific local problems is not its main content, but the establishment of the laws and general properties of numbers by concepts and reasoning is its main content. Of course, this will, in turn, be helpful to specific calculations at a higher level.
The convincing source of theoretical arithmetic: its conclusion is drawn from the concept by using logical methods, and both logical methods and arithmetic concepts are based on thousands of years of practice and the objective laws of the world.
The concepts and conclusions of theoretical arithmetic reflect the nature and relationship of the quantity of things, summarize a lot of practical experience, and show the relationships that are often encountered everywhere in the real world in an abstract form. The objects can be animals, agricultural products and planets.
Therefore, the abstraction of arithmetic is not empty, but through long-term practice, it summarizes some universal properties, which has wide application. The same is true for all mathematics and any abstract concepts and theories. The possibility of wide application of the theory depends on the extensiveness of the original materials summarized in it.
Abstraction has its own limitations: when applied to specific objects, it only reflects one aspect of the object, and often only quantity is not enough. You can’t apply abstract concepts everywhere indefinitely. A sheep and a wolf are added together, and a liter of water and a handful of soil are not the place where arithmetic plus one is applied. Truth is concrete and mathematics is abstract. The application of abstraction to concreteness is often an art and technology.
The development of numbers is also very interesting. At first, it is a number associated with a specific object, then an abstract number, and then a general number. Each stage relies on previous concepts and accumulated experience. This is also one of the basic laws of the formation of mathematical concepts.
The origin and development of geometry is similar to that of arithmetic. The actual needs of measuring and calculating the area of land and the volume of containers, the volume of barns and water conservancy projects have led to the emergence and development of geometry, including the concepts of length, area and volume. For farmers, knowing the area of land is very beneficial to predict the harvest. For water conservancy projects, it is important to know how long the earthwork volume will take to complete the project.
Babylonians and Egyptians were the leaders in the initial years of geometric development (about 3000 BC to 700 BC). At first, geometry is some formulas summed up from experience, including the formulas for finding the area of triangles, rectangles, trapezoid, circles, cuboids, spheres, etc.
The formula A=(8d/9)2 used by the Egyptians to calculate the area of a circle was surprisingly good at that time, where d was the diameter. This formula is equal to taking π=3.1605 from the area formula of a circle. Geometric problems are also arithmetic problems in calculation.
Babylonians and Egyptians should not have realized at that time that their algorithms and rules needed to be based on, or could deduce some conclusions from others through deduction. The formulas or laws they get are not related to each other, so they are not systematic.
At this time, the Greeks appeared. They went to Egypt and Babylon to do trade, travel and study math and science. In this way, the arithmetic and geometry of the Egyptians and Babylonians spread to Greece around the seventh century BC. Then came the era when the stars shone and there were many schools of thought. Interestingly, China was roughly in the Spring and Autumn Period and the Warring States Period, with a hundred schools of thought contending, and many thinkers came forth: Laozi, Confucius, Mozi, Mencius, Zhuangzi, Xunzi and Han Feizi.
The influential schools in the classical Greek period (from 600 BC to 300 BC) are: Ionian School, Pythagorean School, Ernian School, Clever School, Plato School, Aristotle School, etc.
The most important ideological contributions of the ancient Greeks to mathematics include: mathematics studies abstract concepts, and all mathematical results must be deduced by deduction according to the axioms clearly defined in advance.
Geometry thus develops in the direction of geometric theory; Introduce concepts, draw conclusions from experience, clarify the relationship between them, and find new conclusions. In this process, abstract thinking plays an extremely important role. In the spatial form of real objects, geometric concepts are abstractly generated: points (without size), lines (without width and thickness), surfaces (without thickness).
Like arithmetic, geometry comes from practice and gradually forms mathematical theory. Geometric theory studies the abstract forms and relationships of space.
This is different from other sciences that study the spatial form and relationship of objects, such as astronomy and measurement, or art such as painting and sculpture. It is impossible to do experiments in abstract space form, and only logical reasoning can be used to establish the connection between conclusions and derive new conclusions from known conclusions.
The obviousness of geometric concepts, the method of reasoning and the convincing conclusion are all based on thousands of years of practice and the objective laws of the world, just like arithmetic.
When we emphasize the importance of interdisciplinary to scientific development today, looking back at history, we will find that it is a specious formulation.
The intersection of disciplines has been very active in history, which is an important source of further general concepts, methods and theories, and has a great impact on the development of human civilization and science. The greatest scientists, such as Archimedes, Newton, Leibniz, Euler, Gauss and Einstein, have made great contributions in many aspects.
Let’s say that arithmetic and geometry, the earliest two branches of mathematics, were inseparable and influenced each other from the beginning. Simple length measurement is already a combination of arithmetic and geometry. When measuring the length of an object, put a single position of a certain length on the object, and then count how many times it is put together. The first step (placement) is geometric, the geometric concept behind it is congruence or coincidence, and the second step (number) is arithmetic.
When measuring, it is often found that the selected unit cannot be placed on the measured object for an integer number of times. At this time, the unit must be divided, so that a part of the unit can be used to measure the object more accurately, that is to say, not only the integer, but also the fraction can be used to represent the length of the measured object.
The score is thus generated. This is the result of the cooperation between geometry and arithmetic, which produces an important new concept-fraction, and causes the popularization of the concept of number from integer to fraction.
The discovery of irrational numbers also comes from the combination of geometry and arithmetic, but the discovery of irrational numbers cannot be realized by measurement, because the accuracy is always limited in actual measurement, and irrational numbers are infinite acyclic decimals.
Pythagorean theorem tells us that the diagonal length of a square with unit side length is the square root of 2, which is an irrational number. In this way, the concept of number is further developed. Moreover, people gradually understand the number as the ratio of a certain quantity to the quantity taken as a unit.
The discovery of irrational numbers is a typical example that reflects the power and profundity of mathematical theory in revealing natural laws and phenomena. Without mathematics, many phenomena and laws can’t be understood.
The further development of number is the concept of real number, and then the concept of complex number. Then there is the algebraic structure.
Hua Luogeng, the late great mathematician, made an incisive comment on the relationship between logarithm and form: when the number is missing, it is less intuitive, and when the number is missing, it is difficult to be nuanced.
—————————-
1. The original poem: number and shape are interdependent, so how can they be divided into two sides? When the number is missing, it is less intuitive, and when the number is missing, it is difficult to be nuanced; The combination of numbers and shapes is good, and everything is separated; Don’t forget, the unity of geometry and algebra is always connected and never separated! See Selected Poems of Hua Luogeng, China Literature and History Publishing House, 1986.
2. number (sh) number (sh)
Speaking of it, mathematics should start with numbers (sh) and numbers (sh). Who among us can’t count? Soon after we can talk, our parents will tell us to count, and our ability to count will definitely be greater when we get to kindergarten. We usually count
1, 2, 3, 4, 5, 6,……
It seems that most people don’t think of counting all the integers with positive integers. In fact, this is possible, and a number method is:
0, 1,-1, 2,-2, 3,-3,……
In this way, all the integers are counted with positive integers.
Ordinary people should not even think of counting rational numbers (fractions) with positive integers. Intuitively, this seems impossible. Surprisingly, this is also possible.
Fractions can be written as the ratio of integers: 0, p/q, where p and q are positive integers not equal to 0, and there is no common factor greater than one.
First, according to the size of the value of p+q, it is divided into several parts to sort, and each part is counted again, so one number method is:
0,1,-1,1/2,2,-1/2,-2,1/3,3,-1/3,-3,
1/4,2/3,3/2,4, -1/4,-2/3,-3/2,-4,……
In this way, we also counted the rational numbers with positive integers.
Curiosity certainly can’t end like this. We may wonder how to count real numbers with positive integers. This time there is really nothing to do: positive integers can’t count real numbers clearly. This can be strictly proved, but we won’t talk about it here, although it is not difficult.
The story is not over yet.
A question arises here: Is there a set of numbers between the whole of natural numbers and the whole of real numbers, which can’t be counted by positive integers (that is, it can’t establish a one-to-one correspondence with natural number set), and real numbers can’t be counted by this set?
Cantor, the founder of set theory (a branch of mathematics), suspects that such a set does not exist. This is the famous continuum hypothesis. Hilbert gave a report at the International Congress of Mathematicians in 1900, and listed 23 problems, the continuum hypothesis being the first one. This shows the importance of this issue. These 23 problems have a great influence on the development of mathematics in the future.
Godel is a great mathematical logician. In 1940, he proved that there is no contradiction between the continuum hypothesis and the axiomatic system we usually use. Just because there is no contradiction doesn’t mean it is right.
In 1963, Cohen established a powerful method-the forced method. By this method, he proved that there is no contradiction between the hypothesis of continuum and the axiomatic system we usually use. That is to say, in our commonly used axiom system, adding this assumption will not produce contradictions; If this assumption is not added, there will be no contradiction.
This is obviously beyond the expectation of ordinary people. An important and natural question cannot be judged true or false in our commonly used axiomatic system. This shows the strangeness of logic. Cohen won the Fields Prize in 1966 for his work on the continuum hypothesis.
The continuum hypothesis seems to have been understood, but in fact, the thinking on this issue has not stopped, and profound mathematics is still being produced.
We can compare the continuum hypothesis with the parallel axiom of plane geometry. The thinking and research on parallel axioms leads to the emergence of non-Euclidean geometry such as hyperbolic geometry. Riemannian geometry is a kind of non-Euclidean geometry and a mathematical framework of general relativity.
Curiosity, simple good questions can always take us far, far away.
3. Know infinity
In our limited life, it seems to be a difficult thing to know infinity, and it may even be a disturbing thing. The ancient poem "Born less than 100 years old, always worried about being a thousand years old" shows that we are not willing to be confined to our limited time and space.
But infinity is awesome. Pascal said, "When I think of my short stay in life, swallowed up by the eternity before and after, and the small space I occupy, submerged by the infinite and vast space that I know nothing about, I feel scared. The eternal silence of these boundless spaces scares me. "
There are infinite integers and infinite real numbers. In the game of counting, we know that these two infinity are essentially different.
Only mathematics can study the infinite, reveal the magical infinite world, and use the infinite to study the limited. Examples include limit, series, infinite set …
The following two equations can make people feel the infinite magic of mathematical utilization:

—————————-
2.When I consider the short duration of my life, swallowed up in an eternity before and after, the little space I fill engulfed in the infinite immensity of spaces whereof I know nothing, and which know nothing of me, I am amazed. The eternal silence of the infinite spaces frights me. Blaise Pascal, in "pensé es" (originally in French, meaning meditation), 1670.
Hilbert, a great mathematician, has a profound understanding of infinity: "No other problem has ever touched people’s hearts so deeply;" No other thought can stimulate people’s logical understanding of thinking so fruitfully; However, there is no other concept that needs to be clarified more than the concept of infinity. "
4. Some opinions
Great people never skimp on their awe and praise of mathematics:
Mathematics is the core of reality.
-Pythagoras School, Plato School
We often hear the view that "everything is number" comes from Pythagoras, and he has a similar expression: number rules the universe; Number is the essence of everything. Plato School was deeply influenced by Pythagoras School and put mathematics in the highest position: the highest form of pure thought is mathematics.
On the gate of Plato’s Academy, it is written that "those who have no knowledge of geometry are not allowed to enter this gate".
In the seventh chapter of Plato’s Republic, there is a long dialogue to discuss the importance of arithmetic and geometry. The conclusion is that arithmetic forces the soul to use pure reason to lead to truth, geometry is to know eternal things, and arithmetic and geometry are the first and second courses that young people must learn.
Mathematics is the true essence of nature.
-ancient Greece
With this understanding, it seems not surprising that ancient Greece can make epoch-making achievements in mathematics.
Physics is written in the big book of the universe, and it keeps opening before our eyes. But we can’t read this book until we learn to write the characters and language of the universe. It is written in mathematical language, and the characters are triangles, circles and other geometric figures. Failure to understand these means that it is impossible for human beings to understand every word of this book. Without these, people can only wander in the dark maze.
-Galileo
—————————-
3.“Das Unendliche hat wie keine andere Frage von jeher so tief das Gemüt der Menschen bewegt; das Unendliche hat wie kaum eine andere Idee auf den Verstand so anregend und fruchtbar gewirkt; das Unendliche ist aber auch wie kein anderer Begriff so der Adfkl?rung bedürftig.” David Hilbert: In address (4 Jun 1925), at a congress of the Westphalian Mathematical Society in Munster, in honor of Karl Weierstrass. First published in Mathematische Annalen (1926), 95, 161-190 with title über das Unendliche.
Galileo was one of the founders of modern experimental science and mechanical materialism. He established the law of falling body, discovered the law of inertia, and determined the "Galileo relativity principle". He is a pioneer of classical mechanics and experimental physics. He was also the first person who made great achievements in observing celestial bodies through telescopes. Galileo’s view of mathematics can be regarded as a development of the view of the ancient Greeks.
Mathematics is the queen of science.
-Gauss
Gauss is known as the prince of mathematics in the 19th century, the greatest mathematician in the 19th century, and an outstanding physicist, astronomer and geodetic scientist. His words are often quoted, but I don’t know where Gauss took the emperor.
In natural science, mathematics is incredibly effective.
-eugene wigner
Wigner put forward the theory that the nucleus absorbs neutrons and discovered the Wigner effect, so he won the Nobel Prize in Physics in 1963.
This introduction was the topic of Wigner’s report in Courand Institute of Mathematics of new york University on May 11th, 1959. The article was published in the journal Communications in Pure and Applied Mathematics sponsored by Courand Institute of Mathematics in February, 1960. This view of Wigner has a great influence, and the discussion and extension of this view have never stopped since it came out.
—————————-
4.Philosophy (i.e. physics) is written in this grand book, the universe, which stands continually open to our gaze. But the book cannot be understood unless one first learns to comprehend the language and read the letters i n which it is composed. It is written in the language of mathematics, and its characters are triangles, circles, and other geometric figures without which it is humanly impossible to understand a single word of it; without these, one wanders about in a dark labyrinth.” Galileo Galilei, The Assayer (Il Saggiatore (in Italian)), as translated by Stillman Drake (1957), Discoveries and Opinions of Galileo pp. 237-8.
5. "Die Mathematicist Die Knigin der Wissenschaft en und Die Zahlen Theorist Die Knigin der Mathematik", Wolfgang Sartorius von Waltershausen: Gauss Zum Ged Chtnis (Biography of Gauss), 1856.p.79. 。
God is a mathematician of very high rank. He used very advanced mathematics when he built the universe. Our attempt to be weak in mathematics enables us to understand a little bit of the universe. As we continue to develop more and more advanced mathematics, we can hope that we can better understand Universe 6.
-Dirac
Dirac discovered a fruitful new form of atomic theory, so he won the Nobel Prize in Physics with Schrodinger in 1933. The Dirac equation he put forward was hailed as a groundbreaking work, which predicted the existence of positrons and was later confirmed by experiments. The δ function he proposed was very creative and shocking, which was unacceptable in the mathematical theory at that time, but it was very useful in physics.
Later, when the generalized function theory appeared, mathematical theory could explain and deal with the δ function, which turned out to be a generalized function.
Mathematics must control our rational flight; Mathematics is a crutch for the blind. Without it, it is difficult to move. Everything in physics is undoubtedly due to mathematics and experience.
-Voltaire
Voltaire was a French philosopher and writer in the 18th century and a leading figure in the French bourgeois enlightenment. His thoughts represented the thoughts of the whole Enlightenment, enlightened people’s minds and influenced a whole generation.
The strength of French mathematics is not only the achievement of French mathematicians, but also profound cultural factors.
The development and perfection of mathematics is closely related to the prosperity of the country.
-Napoleon
Napoleon was a great French strategist and politician in the 19th century, and the founder of the First Empire of France. People generally pay attention to his military and political achievements. In fact, his achievements in science and education are also crucial to the future development of France.
During the First Empire of France, France established a national education system that has been preserved to this day, and established public middle schools and French universities to train talents and encourage the rise of scientific research and technical education.
—————————-
6. “God is a mathematician of a very high order, and He used very advanced mathematics in constructing the universe. Our feeble attempts at mathematics enable us to understand a bit of the universe, and as we proceed to develop higher and higher mathematics we can hope to understand the universe better.” P. A. M. Dirac: The Evolution of the Physic ist’s Picture of Nature. Scientific American, May 1963, Volume 208, Issue 5.
7. “Mathematics must subdue the flights of our reason; they are the staff of the blind; no one can take a step without them; and to them and experience is due all that is certain in physics.” Francois Marie Arouet Voltaire, Oeuvres Completes, 1880, t. 35, p. 219.
8. “The advancement and perfection of mathematics are intimately connected with the prosperity of the State.” Napoléon Bonaparte: Correspondance de Napoléon, t. 24 (1868), p.112.
Napoleon paid great attention to science and culture. After taking power, he regularly attended the meetings of the French Academy of Sciences, invited academicians to report on scientific progress, and awarded many awards to scientists, including foreign scientists.
Napoleon’s concern promoted the prosperity of French science, and a large number of dazzling scientific stars appeared, such as Laplace, Lagrange, Gaspard Monge, sadi carnot, Fourier, Gay-Lussac, Lamarck and Ju Weiye.
Mathematical science presents one of the most brilliant examples. Without the help of experience, pure reason can successfully expand its territory.
-Kant
Kant was a German philosopher in the 18th century and was considered as one of the greatest philosophers of all times. He has profound knowledge of natural science and a deep understanding of morality. His philosophy has a profound influence on German classical philosophy and western philosophy, and also on the birth of Marxist philosophy. Critique of Pure Reason is his most famous work.
Strange as it may sound, the strength of mathematics lies in its avoidance of all unnecessary thinking and its pleasant saving of mental labor.
-Mach
Mach was an Austrian physicist and philosopher from the 19th century to the early 20th century. The Mach number of high-speed flight is named after him. His most important achievement was that he found the shock wave when he was studying the high-speed motion of an object in gas. Mach’s Mechanics had a profound influence on Einstein. Mach has also been nominated by many people as a candidate for the Nobel Prize in Physics.
Mach’s above viewpoint is a specious truth, which will be explained later by the problem of the seven bridges in Konigsberg and the classification of crystals.
—————————-
9. “The science of mathematics presents the most brilliant example of how pure reason may successfully enlarge its domain without the aid of experience Immanuel Kant and F. Max Mü ller (trans.),’ Method of Transcendentalism’, Critique of Pure Reason (1881), vol. 2, p.610. See also: Critique of Pure Reason, p.575, by Kant, translated by Wang Jiuxing, Commercial Press.
10. “Strange as it may sound, the power of mathematics rests on its evasion of all unnecessary thought and on its wonderful saving of mental operations.” Ernst Mach: in E.T. Bell, Men of Mathematics (1937), Vol. 1, l (Roman numeral ‘l’)).
If I feel sad, I will do math and become happy; If I am happy, I will do math to keep happy.
-Renee
Alfréd Rényi is an outstanding Hungarian mathematician in the 20th century. He mainly studies probability theory, combinatorial mathematics, graph theory and sequence. Renee told us how good it is to do math!
Pure mathematical structure enables us to discover concepts and laws that relate to these concepts, and these concepts and laws give us the key to understanding natural phenomena.
-Einstein
One reason why mathematics enjoys special respect over all other sciences is that his propositions are absolutely reliable and indisputable, while all other scientific propositions are debatable to some extent and are often in danger of being overturned by newly discovered facts. There is another reason why mathematics has a high reputation, that is, mathematics gives precision nature a certain degree of reliability. Without mathematics, these sciences cannot achieve this reliability.
-Einstein
Einstein is the greatest scientist in the 20th century, and is well known to all women and children. Its scientific achievements have changed people’s understanding of the world.
He is not only a great scientist, but also a great philosopher and social activist, deeply concerned about the fate of mankind. The profound understanding of nature, society and human beings makes people marvel at his superhuman intelligence and great heart.
—————————-
11. “If I feel unhappy, I do mathematics to become happy. If I am happy, I do mathematics to keep happy.” Alfréd Rényi: In P. Turán, ‘The Work of Alfréd Rényi’, Matematikai Lapok (1970), 21, pp.199-210.
12. “pure mathematical construction enables us to discover the concepts and the laws connecting them, which gives us the key to understanding nature.” Albert Einstein, In Herbert Spencer Lecture at Oxford (10 Jun 1933), ‘On the Methods of Theoretical Physics’. Printed in Discovery (Jul 1933), 14, 227. Also reprinted in Philosophy of Science, Vol. 1, No. 2, (Apr., 1934), pp. 163-169. For Chinese translation, please refer to the first volume of Einstein’s Collected Works, translated by Xu Liangying and others, Commercial Press, 2010.p.448. 。
13.“One reason why mathematics enjoys special esteem, above all other sciences, is that its propositions are absolutely certain and indisputable, while those of all other sciences are to some
extent debatable and in constant danger of being overthrown by newly discovered facts. … But there is another reason for the high repute of mathemati cs, in that it is mathematics which affords the exact natural sciences a certain measure of certainty, to which without mathematics they could not attain.” Albert Einstein: Geometry and Experience, Published 1921 by Julius Springer (Berlin), also reprinted in “The Collected Papers of Albert Einstein”, Translation Volume 7, Princeton University Press, 2002. For Chinese translation, please refer to the first volume of Einstein’s Collected Works, translated by Xu Liangying et al., Commercial Press, 2010.p.217. 。
The universe is big, the particles are tiny, the speed of rockets, the cleverness of chemical engineering, the change of the earth, the mystery of biology and the complexity of daily use, and mathematics is everywhere.
-Hua Luogeng
Hua Luogeng’s comments on the use of mathematics are extremely incisive.
5. the spirit of exploring the world
In practice, knowledge is gained through sensibility and thinking. Furthermore, through abstract thinking, the connection between knowledge is established and science is formed. At this point, reason and thinking have their own free kingdom.
In one’s own kingdom, thinking often goes far beyond actual needs. For example, some large numbers such as one billion or ten billion are generated on the basis of calculation, and the actual needs of using them are later; Imaginary numbers are generated by solving the equation x2+1=0, and then they are widely used.
Mathematics is concerned with the mathematical laws of quantity and shape, and it is an elf to explore the world. In the free kingdom of thinking, it is dexterous and has a lot of free space to fly, and many achievements will take a long time to be applied after completion. Famous examples include:
The study of conic curve by Greeks more than two thousand years ago was used to describe the motion of celestial bodies in the 17th century.
L Riemannian geometry is the mathematical framework of general relativity.
The role of fiber bundle theory in gauge field theory.
The role of l matrix and infinite dimensional space in quantum mechanics.
The application of probability theory in statistical mechanics, biology and finance.
l ……
Our country’s culture and tradition are pragmatic, focusing on immediate interests. Here, I would like to quote the philosopher Whitehead’s advice:
"For those who limit their knowledge and research to obvious usefulness, there will be no more impressive warning, such as the following example: conic curves have been studied as abstract science for 1,800 years, without any practical consideration except to satisfy mathematicians’ thirst for knowledge. However, at the end of this long abstract research, they were found to be essential keys to acquire knowledge of one of the most important laws of nature."
—————————-
14. Hua Luogeng: "Great use of mathematics", originally published in People’s Daily on May 28, 1959. Reprinted in "Great Mathematics for Use" (selected works of Hua Luogeng’s popular science works), Shanghai Education Press.
6. The wisdom of mathematics
Most people are willing to stay away from mathematics, but Mach said that mathematics can save brains pleasantly (see the previous section 4: Some opinions), which is really confusing. Maybe Mach is talking about the wisdom of mathematics. We use two examples to illustrate this point.
The first example is the problem of the Seven Bridges in Konigsberg. This problem occurred in the 18th century, when Konigsberg was the city of Prussia, and now it is Kaliningrad, Russia. There is a river crossing the city, which divides the city into four parts, and there are seven bridges connecting these four parts, as shown in Figure 16 below.
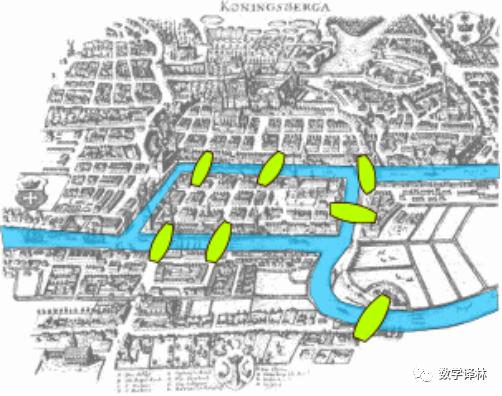
It is said that a popular pastime of the citizens at that time on weekends was whether a route could be designed to cross each bridge just once. No one has ever succeeded, but that doesn’t mean it is impossible. In 1735, the mayor of Danz Creek (about 140 axioms west of Konigsberg) was entrusted by a local mathematician to find Euler. Euler was the greatest mathematician in the 18th century. At the age of 28, he was already famous.
—————————-
15. “No more impressive warning can be given to those who would confine knowledge and research to what is apparently useful, than the reflection that conic sections were studied for eighteen hundred years merely as an abstract science, without a thought of any utility other than to satisfy the craving for knowledge on the part of mathematicians, and that then at the end of this long period of abstract study, they were found to be the necessary key with which to attain the knowledge of one of the most important laws of nature.” A. N. Whitehead, Introduction to Mathematics, London WILLIAMS & NORGATE, pp110-111.
16. Source: https://plus.maths.org/content/bridges-k-nigsberg
Euler thought about the problem like this. The river divides the city into four parts, and the size of each part is not important. What matters is the route design of crossing the bridge. Thus, the land can be abstracted into points, and the bridge can be abstracted into connecting lines 17 between points.
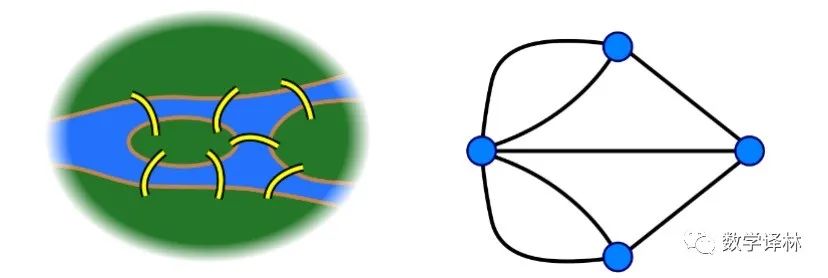
So the problem becomes to design a route on the right picture above, which passes through each connecting line (bridge) exactly once.
Suppose there is such a route. If a point is neither the starting point nor the end point, then the route to this point (that is, the bridge) is different from the route to leave this point. This requires that the number of lines connecting this point must be even.
The picture above has four points, and the starting point and ending point of a line add up to at most two. That is to say, no matter how the route is designed, at least two of the four points are neither the starting point nor the end point, and the number of routes connecting such points must be even. However, the lines (bridges) connecting the four points in the above picture are all odd numbers, namely, 5, 3, 3, 3. This means that it is impossible to design a route for the above picture, passing through each connecting line (bridge) exactly once.
Euler’s way of solving this problem shows the abstract value and the wisdom of mathematical thinking. Euler’s work also marked the birth of a branch of mathematics-graph theory. Graph theory is very useful in information science, including network and chip design.
The second example is the classification of crystals. Diamonds and snowflakes are crystals, which are very beautiful. Crystals have good symmetry. In fact, the symmetry of crystals has a strong constraint on the types of crystals. The branch of mathematics that studies symmetry is group theory. So mathematics has played a great role in the study of crystals. In 1830, German hessel (1796-1872) determined that there were 32 symmetrical forms of crystal shape (called 32 point groups).
—————————-
17. Two sources: https://plus.maths.org/content/bridges-k-nigsberg
After determining the symmetrical form of the shape, people turn to the internal structure of the crystal. Frangen Heim (1801-1869), a 19th century German, proposed that the internal structure of crystals should take points as the unit, and these points are arranged periodically in three-dimensional space.
Later, the French Blavy (A. Bravais, 1811-1863) put forward the theory of spatial lattice, which holds that the center of mass of the material particles in the crystal is distributed at the vertex, face or body center of the parallelepiped unit of the spatial lattice, and the particles are periodically arranged repeatedly in the three-dimensional space. They identified 14 forms of space lattice.
Abandoning all the physical properties of crystals and considering crystals only from the perspective of geometric symmetry, Russian crystallographer Fedorov determined that there were 230 kinds of microscopic symmetry forms of crystals, that is, there were only 230 kinds of spatial (symmetry) groups inside crystals.
Fedorov’s work was the mathematical theoretical basis of the later crystal experiment, which played a great role in determining the internal structure of the crystal. All these 230 symmetries were found in the experiment. In 1912, M.V.Laue, a German, revealed the periodic structure inside the crystal for the first time through X-rays, and confirmed the geometric theory of crystal structure.
Since then, the British father and son Prague (William Henry Bragg, 1862-1942; William Lawrence Bragg, 1890-1971) and Russian Ulf (георгий (юрий) викт). In particular, they measured some crystal symmetries that Fedorov thought were imaginary (that is, symmetries that existed only in theory).
Laue and Prague won the Nobel Prize in Physics in 1914 and 1915 respectively. In the future, there are many works about crystal research that won the Nobel Prize.
7. The beauty of mathematics
Mathematicians, as well as some physicists, have a strong feeling for the beauty of mathematics, and their pursuit for it is endless:
My job always tries to unify truth and beauty, but if I can only choose one or the other, I often choose beauty 18.
-Waier
Weil is probably the greatest mathematician after Poincare and Hilbert in the 20th century, and he also put forward the gauge field theory in physics. His book Group Theory and Quantum Mechanics was first published in 1928.
It is said that theoretical physicists at that time would put this book on the shelf, but they didn’t read it because the mathematics in it was too difficult. Wail seems to believe that beauty is a higher level of truth, because what we see and understand should be only a part of truth.
And beauty can often bring us to a more comprehensive truth.
Beauty is the first test: ugly mathematics has no place in this world.
-hardy
Hardy was an outstanding analyst in the 20th century and the most outstanding mathematician in Britain in his time. His Monologue of a Mathematician expresses his views on mathematics and has a wide influence.
God created the world with beautiful mathematics. When trying to express the basic laws of nature by mathematics, researchers should mainly strive for the beauty of mathematics.
-Dirac
—————————-
18. “My work has always tried to unite the true with the beautiful and when I had to choose one or the other, I usually chose the beautiful.” Hermann Weyl, In Obituary by Freeman J. Dyson, ‘Prof. Hermann Weyl, For. Mem. R.S.’, Nature (10 Mar 1956), 177, p. 458. Dyson notes that this was told to him personally, by Weyl who was “half joking”.
19.Beauty is the first test: there is no permanent place in the world for ugly mathematics. — G. H. Hardy: In it A Mathematician’s Apology (1940) . First Electronic Edition, Version 1.0, March 2005, Published by the University of Alberta Mathematical Sciences Society, Available on the worldwide web at http://www.math.ualberta.ca/mss/. has a Chinese translation: A Mathematician’s Pleadings.
20. “God used beautiful mathematics in creating the world.” “The research worker, in his efforts to express the fundamental laws of Nature in mathematical form, should strive mainly for mathematical beauty.” Paul A. M. Dirac: in Paul Adrien Maurice Dirac: Reminiscences about a Great Physicist (1990), Preface, xv; p.110.
Dirac’s feeling of mathematical beauty is unique. Dirac’s equation is the perfect combination of experiment and mathematical beauty. In Dirac’s view, the equation derived from the experimental results only at that time does not have mathematical beauty, so he modified the equation according to his own understanding of mathematical beauty, and predicted the existence of positrons according to the modified equation, which was later confirmed by experiments. Dirac’s view seems to have something in common with Weil’s.
Dirac should like his equation very much. He first met Feynman at a meeting. After a long silence, Dirac said to Feynman, "I have an equation. Do you have one?". I guess Feynman was very depressed at that time.
Mathematics, if viewed correctly, not only possesses truth, but also possesses supreme beauty.
-Bertrand Russell
Russell, a mathematician and philosopher, won the Nobel Prize in Literature. The History of Western Philosophy, written by him, looks at the history of western philosophy from the perspective of a philosopher rather than a philosopher historian. It has a unique perspective, clear context, smooth writing and no lack of humor. His understanding of beauty naturally has a very broad background.
………
There is no doubt that the meaning of mathematical beauty has some similarities with other beauties such as art in formal beauty, but it is more a beauty of thinking and logic, wisdom and has its own characteristics. Everyone’s understanding of the beauty of mathematics is different, but the following views are helpful to grasp some meanings of the beauty of mathematics:
W form: clear, concise, simple, original, novel, beautiful, the connection between different objects.
W connotation: profound, important, basic and rich in meaning.
W proof: clear, neat and ingenious
Let’s illustrate the above point with some examples.
The first example is Pythagorean theorem, which is called Pythagorean theorem in the west. Hooking three strands, four strings and five is a special case of this theorem, which was put forward by Shang Gao in the early Western Zhou Dynasty. This theorem says that the sum of the squares of two right-angled sides of a right-angled triangle is equal to the square of the hypotenuse:
—————————-
21. “Mathematics, rightly viewed, possesses not only truth, but supreme beauty.” Bertrand Russell, Essay, `The Study of Mathematics’ (1902), collected in Philosophical Essays (1910), pp.73-74.
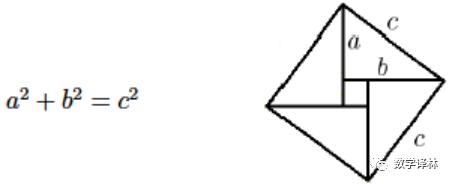
The proof is simple. The area of the big square in the above figure is the square c2 of the hypotenuse, which is equal to the sum of the areas of the four right triangles inside and the area of the small square:
Simplify, expand and gain.
So a2+b2=c2.
The form and proof of this theorem can reflect the meaning of the form and proof part of the mathematical beauty mentioned above. This theorem is basic, and its connotation is profound and rich.
An application of Pythagorean Theorem: On the plane coordinate, the coordinate (x,y) of a point satisfies the equation.
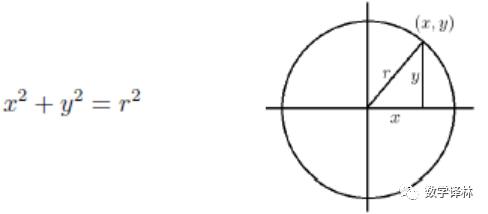
If and only if this point is on the circumference with radius r and the center of the circle is on the origin.
Pythagorean theorem is widely used, which is a manifestation of its basicity. Its profound connotation also lies in that many problems can be derived from it, such as:
What positive integers A, B and C can be the sides of a right triangle?
Is the area of a right triangle with integer sides an integer?
If the sides of a right triangle are all rational numbers, when is the area an integer (for example, 3/2, 20/3 and 41/6 are the three sides of a right triangle, and the area is 5. ) Such integers are called harmonious numbers or congruences.
The third question is closely related to the BSD conjecture of the Millennium problem. Who can solve the BSD conjecture, in addition to honor, can get one million dollars. 157 is a harmonious number, and the length of the three sides of the "simplest" rational right triangle with 157 as the area is:

The complexity and difficulty of the third question and BSD conjecture can be seen from this.
When talking about the meaning of beauty in mathematics, one of them is "the connection between different objects". This seems to have nothing to do with beauty, but it is actually a very important point of thinking, logic and wisdom. We look at Pythagorean theorem from this point of view.
Generally, people look at Pythagorean theorem as follows: knowing the length of two sides of a right triangle, we can find the length of the third side. This pragmatic thinking hinders our exploration and innovation. Looking at it from another angle, Pythagorean theorem reveals the relationship between the three sides of a right triangle.
This angle gives us a broad view at once. For example, the square of three numbers can be related to Pythagorean theorem or higher power:
This is the famous Fermat equation in number theory. Whether they have integer solutions without zero (that is, A, B and C are integers, but none of them are zero) is a problem that has puzzled mathematicians for more than 300 years.
In order to solve this problem, a great mathematics, algebraic number theory, has emerged, and now it is a very active research direction, with many famous scholars. The Fermat equation problem was finally solved by Wells in the 1990s, which was a great mathematical achievement in the last century. It was a sensation and the story behind it was unusually wonderful.
The second example is from Euclid’s Elements, which asserts that there are infinitely many prime numbers. There is a beautiful proof in Euclid’s book: If the conclusion is incorrect, then there are only a limited number of prime numbers, which are set as p1, p2, …, pn. Multiply them all and add 1 to get a number.
m=1+ p1p2 …pn
Then p1, p2, …, pn are not factors of M, so the prime factors of M are different from those N prime numbers. This is a contradiction, so there are infinitely many prime numbers.
This proof is neat and ingenious, which can make people feel happy mentally. Prime numbers seem easy to understand, but they are probably the most mysterious and elusive objects in mathematics. For prime numbers, it is easy to ask some questions that primary school students can understand, but the most intelligent mathematicians for hundreds of years can’t solve them.
For example, how much prime number occupies in natural numbers?
Goldbach conjecture: Every even number greater than 2 is the sum of two prime numbers.
Twin prime conjecture: There are infinitely many prime numbers P, so that p+2 is also a prime number.
The formulation of the first question is not clear enough. We can make the question more clear: how many prime numbers are there between 1 and n for any natural number n. Nobody can answer this question. But mathematicians have made a lot of progress.
At the beginning of 19th century, German mathematician Gauss and French mathematician Legendre put forward a famous conjecture about the proportion of prime numbers in natural numbers. At the end of 19th century, Adama and Dellavalle-Posen first proved this conjecture respectively, which is the famous prime number theorem. In 1949, Selberg and Erdis respectively gave elementary proofs of the prime number theorem. This is part of Selberg’s important work of winning the Fields Prize in 1950.
The second question is easy to understand, and it is easy to give examples, such as
12=5+7, 88=5+83=17+71=29+59=41+47, ….
So far, the best work on Goldbach’s conjecture is still the result of Chen Jingrun. His paper published in 1973 proved that every sufficiently large even number can be written as a prime number plus another number, and the number of prime factors of the other number does not exceed 2 (for example, prime numbers and 6=2×3 are such numbers, but 12=2×2×3 has three prime factors, which is not satisfactory).
Chen Jingrun’s result is known as Chen’s theorem in the world. In China, it has a misleading name: Chen Jingrun proved that 1+2 is a by-product of Xu Chi’s influential reportage "Goldbach conjecture".
Xu Chi’s reportage inspired a generation’s enthusiasm for mathematics and respect for Goldbach’s conjecture. Chen Jingrun also received a huge amount of letters of admiration and affection. This grand occasion never happened to mathematicians again.
Someone once told me about Chen Jingrun’s work, and he understood 1+2 completely literally. I tried to explain to him the meaning of 1+2 in Chen Jingrun’s work. He gave me an oblique look and said, "You don’t understand". I cried speechless and sighed deeply that it was not easy to do popular science.
At the same time, it is also found that sometimes people are obsessed with their own unrealistic understanding, which seems to be inseparable from their self-esteem and mental security.
The third question is also easy to understand. For example, 3, 5 and 41, 43 are all prime pairs with a difference of 2. The question is whether there are infinite such prime pairs.
In 2013, Zhang Yitang, a Chinese mathematician, made a great breakthrough in this issue. He proved that there are infinite pairs of prime numbers, and the difference between each pair of prime numbers is less than 70 million. Zhang Yitang’s result was a sensation, and the story that he kept pursuing his ideal in adversity was also very inspiring and touched the world.
Prime numbers are one of the most basic objects in mathematical research. So far, it seems that human beings have not shown enough intelligence to fully understand them. The most famous problem in mathematics is Riemann hypothesis, which is closely related to the study of prime numbers. In fact, Riemann put forward this conjecture at that time in order to study prime numbers. It is not surprising that Riemann’s hypothesis has not been solved yet.
The third example is the irrationality of the root number 2, which was a number that brought many troubles in ancient Greece. Theorem: If x2 = 2, then x is not a rational number.
We can also give an aesthetic proof. If the conclusion is incorrect, there will be integers a and b so that x = a/b. It can be assumed that a and b are coprime. Square both sides of XB = A, and you get X2B2 = A2. That is 2b2 = a2, so a is even and a = 2p.
So 2b2=4p2 and b2 = 2p2, so b is an even number. Therefore, A and B are even numbers, and there is a common factor of 2, which is contradictory. So x is not a rational number.
Here, maybe we will suddenly think of whether pi, which was learned in primary school, is irrational? It seems that no one in primary school or middle school has talked about it. In fact, this is a good question, which is closely related to the famous problem of turning a circle into a square in ancient Greece.
This question says whether a square can be made only by ruler (without scale) and compass, and its area is the area of a given circle. It was not until Lin Deman proved the transcendence of π in 1882 that the answer was no.
Lin Deman’s work tells us that π is actually an extremely unreasonable number, called transcendental number, which is much more unreasonable than root number 2. The study of transcendental numbers is also very interesting and an important part of number theory. In the last century, Becker won the Fields Prize in 1970 for his research on transcendental numbers.
It is impossible to fully understand the beauty of mathematics without talking about its formal beauty. There are many important geometric objects in geometry that are extremely beautiful and amazing.
(1) Minimal surface: Minimal surface is very important in differential geometry. Minimal surface is the main tool in Qiu Chengtong’s proof of positive mass conjecture in general relativity.

(2) Fractal geometry: Fractal was discovered in the study of coastline in the last century, and later became an important branch of mathematics.

(3) Power system: Power systems are everywhere. The study of dynamic system in mathematics originated from Poincare’s study of three-body motion in astronomy, and now it is a very active branch of mathematics, and many people have won Fields Prize for the study of dynamic system.
—————————-
22. The following four color maps and the graphics in the next section are all from the Internet.

(4) Karabi-Chueh Manifold: Karabi-Chueh Manifold is a very important manifold, which has many researchers and plays a basic role in string theory.
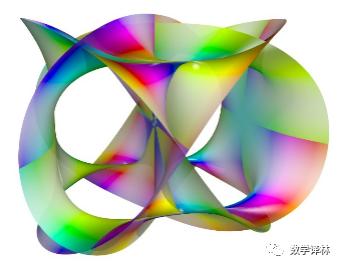
There is no doubt that we can show the beauty of quality and form in mathematics indefinitely, but due to the limitation of space, we should give up this idea and leave more mathematical beauty to readers to explore.
8. Mathematicians
Mathematicians are a group of people with special talents, and their personalities and anecdotes are also colorful.
Weiner, the founder of cybernetics, is moving. On the day of moving, his wife repeatedly told him to go to the new address after work. Of course, as usual, Weiner forgot to return to the old site after work and found something different. I found a girl next to me in the dim light and asked, "I’m sorry, maybe you know me." I’m Norbert Weiner. We just moved. Do you know where we moved? " The girl replied, "Yes, Dad, Mom knew you would forget."
Wiener visited China in 1930s and gave lectures in Tsinghua. He appreciated Hua Luogeng very much.
Deligne was brilliant and won the Fields Prize for proving Wei Yi’s conjecture. He said: Whether you can do math problems is just a psychological problem. This is quite a bit like saying that I can do it, and saying that I can’t do it. This statement also echoes a widely circulated story.
One day, a student was late for a class in a cow university, and the class was over when he got to the classroom. There are seven topics left on the blackboard, which he thinks are homework. He will do these homework when he goes back. A week later, it was time to hand in homework. The student felt very painful. He only worked out two questions. Although he had a good idea for the third question, he had no time to finish it. When he threw his partially finished homework on the professor’s desk in frustration,
Professor: What is it?
Student: Homework.
Professor: What homework?
Only then did the students understand that what the professor wrote on the blackboard last week was the seven most important unsolved problems in this direction. It is said that this student has never done such an excellent job since he became a professional mathematician.
Hungarian mathematician Erdis is legendary, has no fixed residence, always travels, and cooperates with mathematicians there whenever he arrives, so the number of his collaborators is amazing. He believes that mathematicians are devices that turn coffee into theorems.
Siegel, a German mathematician, won the first Wolff Prize. He is a very smart and hardworking mathematician. Hiroyuki, a Japanese mathematician, won the Fields Prize. He often said that he was not talented, but he was meticulous and devoted himself to his work. When he first learned Algebra written by Vander Waals, he almost didn’t understand it, so he began to copy books until he understood it.
A mathematician talked about his late colleague: "He made many mistakes, but they were all made in a good direction. I tried to do this, but I found it difficult to make good mistakes. "
Physicist Kelvin (after whom Kelvin temperature is named) looks at mathematicians like this: Mathematicians are people who think the following formula is obvious:
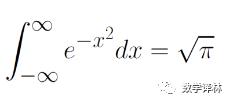
Descartes was a mathematician and philosopher. Mathematically, he founded analytic geometry. Philosophically, he put forward "I think, therefore I am", which caused people to think deeply about the relationship between consciousness and existence.
There is a rumor that he is in love with Princess Christina of Sweden, and the writing communication is blocked by the royal family, so he uses the equation r=a(1-sinθ) to express his passion. The princess quickly understood this unique love letter after reading it. This equation is a polar coordinate equation, and its image is
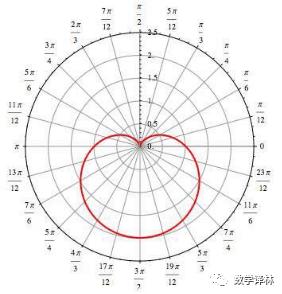
It seems that mathematics is not only a language to describe nature, but also a language to describe love.
This paper is based on the author’s report of the same name. Most of the materials in this paper are well known. The main reference materials for the historical part are as follows:
1. Mathematics, its contents, methods and significance, Volume I, A.D. Alexandrov et al., Science Press, 2001.
2. Ancient and modern mathematical thoughts, Volume I, by M. Klein, Shanghai Science and Technology Press, 1979.
Other references are quite complicated, including network resources, some of which are listed in the notes in the article, and there are still many references that are difficult to list one by one.
Welcome attention
Popularization of science Liaoning
Original title: "Academician Xi Nanhua: The Meaning of Mathematics"
Read the original text